Maths Ext help (1 Viewer)

[Farhanthestudent005]

 

[cossine]

So the integrand is area of the cross section. So just integrate that.

 

[5uckerberg]

View attachment 35850

So okay this is another find the volume of the cylinder type of question.

Step 1 Recognise which axis is the region rotated on.
Step 2 Know the volume of the cone and note that the x-axis represents the height using the concept of the cone through the rotation about the x-axis in
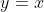
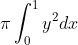
Step 3 Write in because the boundaries are 0 and 1 which is the height thus explaining this part
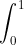
Step 4 Write in
Step 5 Expand
Step 6 Multiply by
Step 7 Integrate
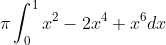
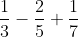
Step 8 Solve by completing the calculation.

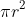
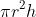
What you will see here @Farhanthestudent005 is that you need to know what the volume of the cylinder is but with a minuscule height because at the end of the day when you rotate a graph along either the x or y-axis or z-axis if you are in 2nd-year uni or above you are inevitably going to have circles and the area of the circle is so then to find the volume of the cylinder you have to have or to recognise the pattern it will be

 

[5uckerberg]

If you have any difficulties feel free to reply to the post and someone will help you along your mathematical journey.